« Part 2 The Modern Era | | »
Part 3. The Advent of Commercial Mechanical Calculation.
| Mini: PHP-GD image library not found. Exiting. | Mini: PHP-GD image library not found. Exiting. | Mini: PHP-GD image library not found. Exiting. | Mini: PHP-GD image library not found. Exiting. | Mini: PHP-GD image library not found. Exiting. | Mini: PHP-GD image library not found. Exiting. |
On this page… (hide)
1. A time of change.
The late modern period, spanning the nineteenth century through the two world wars of the twentieth century and ending roughly in the middle of that century, was a time of enormous economic, technological, cultural and political change. The role of calculators, from one point of view, was a comparatively minor part of this time of panoramic and turbulent change, and yet, it also was profoundly shaped by it, and helped facilitate its development.
As already suggested, none of these devices that were created during this period sprung from their inventors’ minds completely unprecedented. The roots spread back into the early Modern period from the earliest forms of embodied calculation (for example, the use of calculi). The period following the inventions already mentioned of Schickard, Pascal, Moreland and Leibniz were followed by a multitude of devices built on similar basic principles, but all with the limitation that they were not widely taken up because as yet need had not developed to resonate with the limited capabilities and often high cost of the inventions. Nevertheless, as time passed a web of developments would continue to emerge that would eventually create that moment when such a resonance might take place. Central to this was the use to which these devices might be put.
Usefulness arises in a context, and the social, political, economic and technological context was in a process of unprecedented transformation across Europe. The late C18 was characterised most dramatically with the execution of Louis XVI, in 1793, by a transition between the power of the ancienne regime to that of new political forces. Into the following century across Europe the emerging power of merchant and market increasingly swept aside the old aristocracy in the shaping of the politics, economics and infrastructure of what, in a process evolving from the Treaty of Westphalia (1648) had emerged as ‘sovereign’ nation states. Improving systems of transportation facilitated a process of ever intensifying trade across and between states was involving monarchs, peasants, artisans, merchants and financiers alike.1 Ever more efficient processes of printing and dissemination facilitated production and dissemination of ideas. A period of comparative peace between nations in the late C18 was marked by a revolution in agricultural production. But this was just one way in which a synergy between scientific and technological innovation was finding purchase within transforming systems of production, commerce, governance and more broadly lived experience. In addition, science was beginning to be harnessed to actual production. Steam replaced horse, and then from the mid C19 to the mid-C20, electrical networks spread across Europe and then much of the rest of the modern world allowing the introduction of many new technologies.
Accompanying this the understanding of the world, and even the conception of meaning in existence, was being transformed in conjunction with the emergence of new scientific insights in mathematics, physics and astronomy. As Werner Heisenberg, a founding physicist in quantum mechanics has summarised it:
Not only was the conception of nature being transformed through a more technical, and indeed mathematical account, but new literacies and cognitive skills were now required. Skills were now more broadly required to deal with an economy that was ever more shaped by the market, production that was ever more shaped by science, and products whose use required continual processes of cultural learning. Mass education in reading and basic mathematical skills were now an increasing necessity. Steadily the period of “childhood” was being extended (including, eventually, the invention of the “teenager”) to allow an extended period of socially conceded time in which this personal development could take place.
Finally, technological development and the role of science was greatly accelerated by the two world wars, which became wars of science, as evidenced by leaps in aviation, rocketry, electronic communication, encryption, and detection - to name just a few - and cumulating in the harnessing of the process of nuclear fission itself to bomb Hiroshima and Nagasaki. Pressed forward by the onward thrust of market and military priority, the systematic research, development and deployment of new techniques, powerful precision tools, and diverse materials, often emerging in new goods, produced through evolving processes of mass production and social organisation, became a constant theme of the times.
It was then in this remarkable period of dynamic change that the insights into calculation technology finally found purchase.
2. Simple devices
As the above suggests, historical periods do not easily fit together as simply defined blocks of time with nice clear boundaries. Rather they are useful labels for different times of change, which whilst usefully distinguished, overlap each other to allow for the transitions which take place across them. The process of technological change in particular, in any particular period, is layered over, and has roots reaching back to the innovations which precede it. Indeed, not only did prior inventions provide a base on which new more commercially successful devices might be built, but also old methods could be persistently found in use sometimes right through the late Modern period. One of the most common of these, which may still be found in use in some places, was ready reckoners.
2.1 Ready reckoners
Ready reckoners were in widespread use by shopkeepers and many others from the C17 on. They presented tables of precalculated results of many kinds of multiplication and division, addition of fractions, important constants, unit conversions, logarithms, and much else. Frequently they included the much needed calculations of the price for multiples of an item for sale, or per unit of weight and could also be used to look up the calculations needed for wages and interest and .3 One such, from 1892 is “Ropp’s Commercial Calculator: a practical arithmetic for practical purposes, containing a complete system of useful, accurate, and convenient tables, together with simple, short, and practical methods for rapid calculation”, published in the US and used widely into the early C20. This one, shown below, is the 1892 World’s Fair Edition containing 128 pages of useful mathematical facts, formulas and tables.

1892: Ropp’s Commercial Calculator
(collection Calculant)
The dominant role of ready reckoners in this period is indicated by M. Norton Wise’s comment in relation to Victorian England that:
2.2 Lookup tables in new form
Other early new mathematical devices to gain broader use were simple adaptations of the sorts of tables that might be found in ready reckoners. There was an ever more extensive need to be able to measure and compare especially in the expanding processes of trade and commerce. The bane of earlier centuries (and even still) is lack of standardisation across localities in which trade took place. In particular, quantities were often not measured in the same units. As a result, special purpose devices, basically just forms of mechanical look-up tables were devised to enable conversion, needed not the least since performing associated conversion calculations each time was not only time consuming but beyond the skill of many who might need them. A particularly stark need for such devices was created, following the French Revolution, when the Revolutionary Government introduced metric measures. A "Convertisseur" from (1780–1810) designed by clock maker Gabrielle Chaix in Paris to assist in converting between the old measure of distance (the "Aune") to the new metric metre that was introduced in 1791, is shown below.

~1790: Conversion device
(collection Calculant)
The resemblance between the design of the above and that of a prestigious gentleman’s pocket watch is probably not coincidental. Mechanisation can come in the most basic forms and still carry the allure of the new whilst usefully substituting for otherwise necessary mental skill. Many conversion devices of similarly simple construction continued to be manufactured into the second half of the twentieth century.
2.3 Available principles
It is useful to recapitulate the available principles which had been discovered upon which devices for more sophisticated arithmetic tasks could be devised. Key to all of these was the idea, already embodied in calculi and counting tables, or the abacus, that addition of numbers represents the addition of units of something physical. Bearing this in mind, adding units of motion in space could do the same job, whether the movement was units of:
- movement of a point along a line (implemented for example by a sliding rod, moving chain or strap, or something similar)
- rotations of wheels and cogs
- something else that can be moved (for example, although it was barely used,5 the height of columns of water).
Subtraction could be achieved by units of motion in the opposite direction, or where that was not possible, the addition of complementary numbers.
Multiplication could be achieved by repeated additions, and divisions by repeated subtractions. Alternatively tables of multiplication (or logarithms) could be utilised to replace these steps.
Examples utilising these analogue motions to aid arithmetic were already available from as early as the C17.
- The slide rule had used the motion of sliding along a straight line to add distances representing a logarithmic scale.
- The devices of Schickard, Pascal and Leibniz had used rotation of dials to achieve addition of rotations. Schickard’s machine had the capacity to subtract by turning the dials backwards, but had not fully resolved how to carry from one dial to another.
- Pascal had developed a very efficient carry mechanism but at the expense of the dials not being able to be rotated backwards. Both of these relied on a stylus for input.
- Leibniz had developed his step drum to improve the input process and repeated additions were facilitated by the capacity of the machine to be turned with a crank handle. But his carry mechanism needed further development to work when this occurred.
It would be tempting to present the subsequent development devices as a single linear track of improvement from these promising beginnings more than a century before. But this would be artificial. Rather a genealogical tree of innovations spread out from these earlier inventions as a niche for their use began to be uncovered.
2.4 Simple linear devices
One such line of development was based on the possibility of adding linear displacements of strips of metal. This type of device, first developed in the 17th Century by inventors such as Claude Perrot (1613–88) was popularised by J. Louis Troncet, a French inventor who created his Arithmographe, later to be known as the Addiator (of which there is one in this collection). A bewildering number of different designs around this idea appeared in the late C19 and early C20. Some used strips or rods, and some used chains which were more versatile. A rather beautiful Locke Adder which uses strips moved by knobs, from 1905–10, sold by Clarence Locke, is shown below.
 |
| Locke Adder 1905–10 (collection Calculant) |
Other simple linear devices in this collection are shown below. (Clicking on the image will take you to a larger image on the description page.)
The above relied either on the movement by a stylus, or knobs, of strips, rods or chains to achieve an addition. Some (such as the Locke adder) used a simplified form of complementary arithmetic for subtraction. Others, such as the Golden Gem, allowed reverse motion for direct subtraction. Others, such as the Addiator, could be turned over to perform the subtraction on the reverse side. Most (but not the Locke Adder) had a simple provision for carries. And some (such as the Scribola (which also had a very early approach to printing its result on a paper strip) had clearing levers to bring its display back to zero for the next calculation. All however, whilst affordable, were cumbersome and slow to use since each digit had to be individually entered by performing a sliding motion. It was much quicker to add the numbers on paper using mental arithmetic. But, of course, where that capability was lacking, or where it was insufficiently well practised and the effort of doing it was consequently considered tedious, then these devices began to find a market in the first half of the C20. Thousands were produced and sold across the more industrialised countries of Europe, the British Empire and the USA.
2.5 Simple rotational devices
Similar simple devices were developed which utilised simple rotation of cogs, with a crude carry process (usually along the lines of that used by Schickard). They relied for their success on having but a small number of interacting cogs, so that the carry mechanism would not jam, or adopting the use of springs (in place of Pascal’s weights) which would store rotary motion to then be utilised when a carry was required. The device shown below was the first model made by C.H. Webb (from New York) who began marketing it in 1869. It is made of brass and is set on a heavy wooden base.
The most obvious other development beyond the subtleties of carry mechanism was the developments in the use of materials. Here we see the heavy two wheel mechanism of the Webb Adder, in brass and wood, counterposed against the SEE Adding Machine which is entirely composed of plastic, has a Pascaline-like weighted carry mechanism, but is built so lightly utilising a materials evolution over three centuries, that a much simplified approach was by now implementable.
A particularly elegant, if made of brass and thus heavy, device was the Swiss Conto (see above) which had not only knobs to enable numbers to be input with a quick turn (rather than requiring stylus input), but also an efficient carry and clearing mechanism.

Webb Patent Adder and Talley Board 1869 |
Other simple rotational adding devices in this collection are shown below. (Clicking on the image will take you to a larger image on the description page.)
(collection Calculant - all above)
The main advantage of these devices was that they were cheap to manufacture and could be easily mass-produced. A lot of innovation is evident in the detail of these machines. A particularly simple approach to the issue of carrying was addressed in the Addall from 1910, a circular proportional adder which used rotations of a wheel to wind on successive numbers whilst a ball bearing, moving in a spiral groove, tracked the successive rotations. The Calcumeter from the same period uses a spring (instead of gravity as with Pascal’s sautoir) to capture the potential energy from rotating an input wheel, releasing it when a carry is required as the wheel passes from 9 to 0.
2.6 Early keyboard adding machines
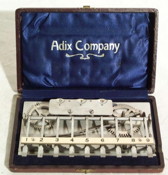
Early experiments with keyboards also were evident in the late C19, some of which are shown below. (Clicking on the image will take you to a larger image on the description page.) Each of them is very different in appearance yet all four - the Spalding Adding machine of 1884 (of which only a very few were produced, and fewer still survive), the Centigraph of 1891 (also extremely rare), the more conventional looking layout of the Addix Adding Machine of 1905, the similarly conceived Adder of 1908, and the Kuli of 1909 - all had a similar principle of operation. In each case the depression of the different number keys actuates a lever to move a gear wheel a corresponding number of notches, thus adding the number. Each of them of course achieves this in their own way. And they have very different appearances with quite different forms of visual output of the accumulated number - dials (for the Spalding), a two digit display and arrow pointing out the 100s (up to 500) for the Centigraph, and a more conventional set of windows for the Addix and Adder indicating that there are many ways that a simple basic principle can be expressed. Each has the rather severe limitation of only being able to add a single digit (from 1 to 9 in the case of the first three). The Adder whilst quite similarly limited has the innovation of a “10” key enabling addition in the middle column. More importantly it is equipped with a clearing key enabling the three windows of the result register to be reset simultaneously to zero. None of these devices, however, was particularly useful given their clumsiness of operation (for example, in clearing a result, with the exception of the Adder), and limitations in function (essentially to addition of a single integer between 1 and 10).
The Kuli, first marketed in 1909 as a further development by the Addix Company, represented a transition from these machines to something potentially much more useful. It had a similar keyboard to the Adder including a “10” key which enabled numbers from 1 to 99 to be entered directly and a highly functional clearing crank handle. In addition, it also had a moveable carriage so that much larger numbers could be added by moving the carriage to the right to successively enter additional numbers of hundreds, thousands, etc. In principle this enabled multiplication and even division to be done on it, albeit with all the tedium of doing that by hand. The paper strips provided were intended to allow the user to write down intermediate results in support of this process. However, large and comparatively expensive as the machine was, its utility was not great enough to earn it any significant commercial success.

Spalding Adding Machine 1884 |
(collection Calculant - all above)
All of the above machines, whether based on rotating gears or lever mechanism had their limitations. As they became cheaper and more accessible not only to shopkeepers but ordinary citizens who might value the assistance they offered, for example in their role as taxpayers,6 these devices became increasingly popular. However, they suffered from the same problems of clumsy input and slow operation that afflicted the simple linear machines. Some such as the Adall could only add, others had no provision for zeroing, and all were slow to use. Further, such adders, whilst simple in concept and cheap to manufacture, were so slow to use for repetitive calculation that, with the partial exception of the Kuli, they were virtually useless for the key arithmetic operations of multiplication and division. But given multiplication could only occur on the Kuli through repeated entry of a number even there it did not really provide a practical method. A quick method for repeated addition and subtraction was required at minimum to achieve these other two functions. That required a more expensive approach and a much more elaborate machine, the first commercial variant of which was designed and sold as the “Arithmometer” by Thomas de Colmar.
3. Commercial “four function” calculating machines
3.1 Arithmometers
Thomas de Colmar first made public his design for a calculating machine capable of doing all four functions (addition, subtraction, multiplication and division) when he was granted a patent for it in 1820.7 His initial design was very different from the machines that would ultimately become the first successfully commercialised four function calculators, half a century later.8 The first design consisted of four step drums (along the lines invented by Leibniz, but not necessarily based on having ever heard of, let alone seen the internal mechanism of Leibniz’s invention).9
Whether or not Thomas reinvented the step drum, even in this first design, the four integers to be added (or subtracted) were set by sliding four corresponding cogs to the appropriate position on such drums. The cogs were moved by sliding nobs along four (square section) axles which were in turn attached to output dial wheels which showed the correct number through small windows. A carry mechanism was incorporated. Rather than being turned by a crank handle the stepped drums were turned by a ribbon that was pulled out from the side. All later designs used a crank handle to turn the drums as is shown in the diagram by Franz Reuleaux in 1862 shown below.
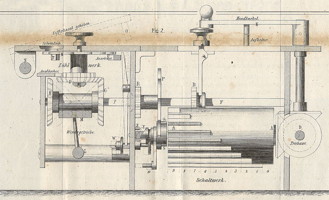
1862: Diagram of the Thomas de Colmar arithmometer mechanism.10 |
The diagram shows a crank handle on the right top which drives the rotation of the step drum (shown centre right). In front of the crank handle can be seen a “slider nob” which moves the cog along the axle to engage the correct number of teeth of the step drum (corresponding to the number to be added). To the left is a reversing mechanism connected to an output dial. The reversing mechanism allows the output dial to be rotated in the opposite direction, if the nob that activates it (situated above) is shifted from the “addition” to the “subtraction” position (where an internal gear reverses the internal rotational motion).
Immediately below is a picture of a step drum from a later arithmometer, but based on the Thomas mechanism, showing the slider, drum with its ‘counting gear’ positioned for the input of ‘5’. In this arithmometer the input number selected shows in the immediately adjacent window. Note the square section axle on which the counting gear moves. This allows the counting gear to engage the axle whatever its position along it.

Step drum (in a later ‘TIM’ arithmometer) |
The Thomas arithmometer was arranged so that the input mechanism could be shifted in relation to the output dials. In this way it was possible to carry out “long multiplications” (by repeated additions) or “long division” (by repeated subtraction). However, in the early decades the machine was not particularly reliable. In particular, the Thomas arithmometer did not really have a reliable carry mechanism until a patented mechanism was introduced in 1865, and this remained the fundamental system for carry in successive generations of arithmometers for the next 50 years.
As with previous calculating machines, commercial success for the arithmometer machine was far from assured. The high finish in lacquered brass and ivory, heavy brass mechanism in the arithmometer’s design still reflected the high status market it was initially seen as appealing to. Thus, it could have become just another prestigious mathematical machine in the cabinets of curiosities of those of elevated status. The somewhat circuitous route to its later success is described by Stephen Johnston.11 As Johnston notes, Thomas did not himself make these early machines. The first of his machines to survive was made by in Paris by Devrine, as usual a local clock (and instrument) maker.
Thomas de Colmar himself was the director of an insurance company. In the 1820s the insurance industry was rapidly expanding as a consequence of free trade and the transport revolution, and France had become an international centre of the industry.12 The likely consequent intensification of work no doubt galvanised Thomas’s interest in finding an efficient way of handling multiple calculations. But given his responsibilities in an intense commercial environment is unlikely that he would have been able to spend all or even a great deal of his time on designing and building more arithmometers over the next several decades. Indeed it was not until several decades later that, in 1844, the arithmometer, very much re-designed, appeared at a French national exhibition. There it could be found amongst precision instruments in a category of 'diverse measures, counters and calculating machines'.13 Johnston thus argues that far from being a lone pioneer, the machines of Thomas were in competition (and regarded initially as a poor second) to others. However, over a half century, the machines of Thomas, as they evolved, prevailed.14 Johnston notes that to achieve this “Thomas campaigned both through the press and in commissioned publications. He also engaged in the rituals of patronage, rituals that we might more readily associate with the decoratively elaborate calculating machines of the 18th century.”15 However, the design of the Thomas machine was sufficiently beautifully executed to make it an appropriate gift to persons of high standing, a property essential to its successful promotion.
Indeed the Thomas arithmometer did take off as a consumer product in France, the UK and Europe and variants continued to sell right up to the first world war. They were not cheap. For example, in 1872 British engineer Henry Brunel wrote that “I have just got what my mother irreverently calls 'a new toy' – to wit a calculating machine price £12 which does all the common operations of arithmetic viz addition, multiplication, subtraction & division in the twinkling of an eye. It is really a very useful article worth its weight in brass.”16 In terms of today’s purchasing power, £12 from 1872 was the equivalent of £5,840 (~US$8,900) in 2013 (based on average earnings).17
Not surprisingly, the commercial background of Thomas de Colmar gave him a head start in knowing how to manage, promote, and sell his product, and that, must in part be the clue to its success. By the turn of the century Thomas de Colmar arithmometers found homes in science laboratories (particularly astronomical observatories), insurance and engineering companies, and government departments (especially those dealing with finances).
By the time Thomas died, in May 1870, some 800 arithmometers had been made. His son, Thomas de Bojano then took over manufacturing. Thomas de Bojano died in 1881 and Thomas’s grandson, the Compte de Ronseray, continued manufacturing arithmometers under the management of Payen. By 1878 some 1500 Thomas arithmometers had been constructed. Some 180 Thomas arithmometers are known to have survived to the present, of which 110 are in public collections and 50 in private collections. Below is the Thomas Arithmometer in this collection, which is from 1884.

1884: Thomas de Colmar Arithmometer |
The arithmometer in this collection demonstrates the high level of usability that the firm of Thomas had now achieved. It allows the multiplication of two numbers each as large as 9 million and the result can be read out to 16 places. Ivory markers are provided for the decimal point (and to delineate hundreds, thousands and millions for ease of use). The clearing and carry mechanisms are entirely reliable. Indeed, still bearing its original varnish, this particular machine operates reliably some 125 years after it was made. Indicating that its owner was serious about its use in practice the machine appears to have been re-mounted by the famous instrument maker, Stanley, (sometime after 1900) in a better quality and stronger box fixed to a cast iron frame. This allows the machine to be tilted at a suitable angle for easy use. As the only surviving Thomas known to have this improvement, this machine seems to stand as a precursor to later innovations under Payen when he added a (rather less robust) hinged tilt base to his machines. Indicatively, the zero numbers were all painted red, presumably as a precaution against the evident danger that, in actual use, arithmometers would not be properly zeroed prior to a new calculation being commenced.
The repairs carried out on this machine were not uncharacteristic of what was required to keep the arithmometer in reliable working order. The machines however were subject to breakage and expensive to repair. The market for such an expensive machine was quite limited, and despite its uprecedented success, as Johnston concludes, Thomas’s work on the machine still fell “more into the category of vanity publishing than mass production”.18 Nevertheless, it formed the standard against which improvements were sought and new designs contemplated.
The time was ripe for others to attempt to produce improved machines. The Thomas concept was developed and improved by a number of other engineers and marketed from different countries.19 From 1880, other European manufacturers - Burkhardt, Layton, Saxonia, Egli, Bunzel, etc entered the market. The three arithmometers from this collection, shown below , are products of this now enlarging set of competing manufacturers and designers.
(collection Calculant - all above)
The three arithmometers (Arithmometers in this collection demonstrate the sorts of improvements to the basic arithmometer design which now emerged. The first, a TIM ("Time is Money" ) arithmometer manufactured by Ludwig Spitz and Co. of Berlin-Tempelhof in 1909 was greatly strengthened from the Thomas design to create a much more robust and reliable machine. First produced in a wooden box it was later made much stronger by installing it in a cast iron frame which held all parts rigidly.20 Beginning a change in style that would be emulated in many other machines the brass panels were now enamelled black consistent with the management, industrial and commercial settings where it was intended to find its market. The other two machines in this set would also originally have had a similar black finish. (Unfortunately, there was a tendency in the late C20 to “take the machines back to brass” in the same way that in the 1960s there was a trend to remove the original finish from wood on vintage furniture, and plaster from brick walls in old houses.)
Burkhardt in Austria produced the first Austrian version of the Thomas machine, and later improvements were marketed in association with Bunzel. Hugo Bunzel, a former caligraphy teacher in Prague, designed the machines that became known as the Bunzel-Delton. The were manufactured by the Bunzel- Delton-Werk Fabrik automatischer Schreib-und Kechenmaschinen in Vienna.21 The second machine above is of interest since it is a one of its kind prototype made by these workshops. It shows the “arms race” that was now underway between competitive designs. Already another radically different design of calculator (the pinwheel described below) had emerged to compete with the arithmometer. Its crank handle was mounted on the side of the machine, making it much easier to turn than the top mounted handle of the traditional arithmometer. The second arithmometer in this collection above seems to encapsulate a patent filed by Bunzel on May 25, 1914, for enabling the position of the crank to be shifted from its normal position standing upright on the deck to one where it is horizontal on the side of the machine mimicking the convenience presented by the pinwheel. Indicative though this is, the prototype was never put into production.
A much sought after goal for calculator designers was to develop a method which would completely automatically carry out division. All the previous arithmometers required a process of “long division” where the divisor was subtracted until no more subtractions were possible. However, this required the operator to either guess when this point had been reached, or subtract one time too many (creating an “overflow”) and then reversing the subtraction to get the correct outcome. A race had been on to try to solve this particular problem, and in 1901 Alexander Rechnitzer filed a patent in the US Patent Office with a solution. In essence it involved a mechanism that could determine that an overflow had occurred (indicated by the most extreme left hand dial going from 0 to 9). When this occurred the mechanism advanced the carriage one place and added the subtracted number back one time, thus setting the machine up for the next partial division to take place.22
The above principle was incorporated into a MADAS arithmometer, developed by Erwin Janz and manufactured by Egli in Zurich, starting in 1913. The acronym MADAS was short for "Multiplication, Addition, Division - Automatically, Substraction"). The achievement of an entirely automatic division process - the first ever for an arithmometer style calculator - was a considerable triumph. In this collection there is a MADAS IX Maxima calculator (produced in 1917), an ambitious machine also in that it could accept 9 digits, up to one thousand million, as input and could display 16 digits (representing numbers up to ten thousand trillion) in its results register. Two MADAS models were produced and by 1920 about 1,000 were being produced with some 7,000 having been sold from countries as wide apart as Europe and Australia.23
3.2 Pinwheels
The pinwheel calculator was developed as an improvement on the arithmometer more or less simultaneously in Russia in 1878 by Swedish engineer Willgodt Theophil Odhner (1845–1905) and in the US in 1874, by Frank Stephen Baldwin (1838–1925). Although they worked independently of each other their machines were so similar that the ‘Ohdner’ or ‘Baldwin’ machine became more or less interchangeable terms.24. The designs utilised a clever 'counting gear' in which the number of teeth protruding could be adjusted by sliders (and later a push-down keyboard). A pinwheel and the internal mechanism (with some pinwheels removed) from a Walther pinwheel calculator in this collection is shown below.
 |  |
| A single pinwheel | Mechanism of the Walther pinwheel calculator (parts removed for demonstration) |
| (collection Calculant) | (collection Calculant) |
As can be seen above, the pinwheel (left, above) is a thin disk with the slider shown protruding in the top right corner. It is in the ‘3’ position, with the cam slot rotated pushing 3 teeth out (seen between and behind the fixed teeth at the bottom). These then are the teeth that are added by the counting wheel. (Click on the image to enlarge.)
These pinwheels thus replaced the much larger and more cumbersome step drums of the arithmometers. Thin as these were, and able to be nestled side by side in a compact manner (right, above), these allowed the calculators to be lighter and much more compact than the arithmometers. The crank handle was conveniently on the right end of the machine, and could be turned either forward or backwards (corresponding to addition or subtraction). The output wheels were set in the base, and as with the arithmometer, the input mechanism (including the pinwheels and sliders) could be moved parallel to it allowing long multiplication and division to be carried out.
Ohdner began manufacture of his machines in 1886 under the name “Original Ohdner” but sold his rights also to Grimme, Natalis and Co., Braunsweig, who began marketing the machines under the name Brunsviga.25 In turn they also licenced agents such as Schuster to sell them. Reflecting a later start these machines were made from a wider range of materials than the arithmometers, including iron and nickel alloys as well as brass and steel. The Brunsviga was a quick success selling 20,000 units between 1892 and 1912.[26 Below is the very early Brunsviga-Schuster pinwheel calculator from 1896, followed by the other pinwheel calculators in this collection.

~1896: Brunsviga Schuster Pinwheel Calculator Serial 3406 (collection Calculant) |
(collection Calculant - all above)
As the range of dates for the set of pinwheel calculators in this collection suggests, the pinwheel design was highly successful and capable of being improved on for the better part of three quarters of a century. The second Ohdner in the collection is from 1938, and although possessing some improvements (such as the capacity to move the carriage back and forth with tab keys), was little different in concept. The same can be said for the Facit calculator from around 1945, and even from the 1950s, near the end of the production of such machines, despite its more modern finish and use of plastic fittings (and some improvements such as clearing levers), the Walther 160 remains quite similar in operation to the Brunsviga of 1896, which, significantly, still works as smoothly as the Walther.
The main progress that had been made was thus not so much in functionality as in production methodology. This is epitomised by the Walther 160 and later Walther calculators. Karl Walther’s ancestors made rifles and in 1858 he established his own hunting and shooting rifle company.27. He developed then into pistols including the famous Walther PP series military pistols, production of which began in 1928. They proved to be a worldwide success. However, the second world war in 1945 left his son Fritz with 80 patents and little else. He was able to rebuild the business both in relation to weapons, but also by diversifying into making office machinery, and in particular calculators. By the 1950s he had four large factories equipped with advanced machinery and technique.28 The Walther 160 was by now a classic product of modern manufacturing technology, mass produced, comparatively lightly made, and accessibly cheap. As a result many thousands of them were sold. By 1970 the Walther Office Machines company (Walther Büromaschinen GmbH) employed 2000 staff and was producing almost 120,000 machines per year, with about 50% for export.29
There were of course many variations on this theme. These included the “Frieden Wheel” which managed to achieve the same effect as the pinwheel but through simply a nicely timed use of a cam. More of that sort of mechanical detail for all the machines referred to here can be found in the classic book by Martin,30 and the marvellous websites of Rechnerlexikon31 and John Wolff32.
3.3 Proportional Rack Calculators
One helpful development in design was the development of a proportional rack which moved cogs through a series of increments depending on the length of a toothed rack with which they engaged. Shown below is the rack mechanism of such a calculator. Note how successive racks have moved increasing distances as the crank handle is turned (creating the diagonal pattern). The loose cogs seen on the square section axle are an example of the nine such cogs under each column of keys. Normally lying between the racks when a key is depressed it moves the corresponding cog sideways on the axle to engage with the appropriate rack for the number of the key depressed.

Rack mechanism of a Mercedes-Euklid 29 calculator. |
In 1906 Christel Haman founded the Mercedes-Euklid company who adopted the rack mechanism. In 1910 he incorporated the mechanism patented by Alexander Rechnitzer, referred to earlier, which enabled his machine to automatically perform the process of division, thus becoming the first mass produced machine with this capacity. The fully working Mercedes-Euklid 29 can be seen below (left) and a demonstration Mercedes-Euklid (model 29 from 1934) can be seen below (right).
 |  |
| 1923: Mercedes-Euklid Model 29 calculator | 1923: Mercedes-Euklid Model 29 Demonstration calculator |
| (collection Calculant) | (collection Calculant) |
3.4 Comptometers
A further approach to the problem of the four functions is found in the American invention by Felt, of a device that he called the comptometer. He patented it in 1887. It performs the task of addition by a system of keys and levers. The keys press down rods which, from key 1 to key 9, increase incrementally in length for each successive key. This difference in displacement, magnified by a lever turns an accumulating gear through 1 to 9 teeth as appropriate.
Notably no crank handle is needed. The small force required for the addition comes simply from the key press. The process of multiplication is done by multiple presses of the appropriate key. This is fast, not the least because with two hands up to ten keys can be pressed at once. But subtraction must be carried out by addition of complementary numbers. Carrying of "tens" is implemented between the accumulating read-out wheels.
Felt first demonstrated this famously with a model constructed in a wooden macaroni box in 1885. He patented his design in 1887 and began selling it from his manufacturing company in Chicago. In 1890 he gave it the name “the Comptometer” and energetically promoted it.33 Below is the Comptometer in this collection - an example from 1896 of the first model, cased in wood, and one of the 40 oldest known to still be in existence. Remarkably with a drop of oil on the springs it still works perfectly.

1896: Felt and Tarrant Comptometer |
The comptometer was a highly successful innovation and was sufficiently cheap and useful to reach a broad market. Characterised by extremely effective marketing and capable of being mass produced the device continued to be marketed for some 80 years. Unusually for such innovations it left its inventor extremely wealthy. By then its essential mechanism was being utilised by more than one company. Shown below is the remaining comptometer in this collection, this time made of plastic in the 1950s by the British Bell Punch company. Also shown is a demonstration model showing the keys and lever system used in the Bell Punch machine.
| Date | Description | Maker | Type | Device (click for greater detail) |
| 1950s | Bell Sumlock Comptometer | Bell Sumlock | Model 909/S/117.878 |  |
| ~1955 | Bell Punch Sumlock | Bell Punch | Demonstration Comptometer |  |
(collection Calculant - all above)
3.5 Curta: The peak of miniaturisation (1947–1970)
Finally there is the last and most beautifully miniaturised of the four function manual mechanical calculators. They were designed by Curt Herzstark. Curt was born in 1902 as the son of Samuel Herzstark who had established a calculator manufacturing company in Vienna in 1905–6. Curt’s father died in 1937 but by then Curt was both Director of the company and a highly competent designer in his own right. He recognised the need for a miniaturised four function calculator which could be carried in an engineer’s pocket. He began to design this as a cylindrical calculator which could be held in one hand and operated with the other. It could utilise the modern new light weight alloys of aluminium, magnesium, etc. By the end of 1937 he had the form of what he wished to accomplish firmly in his mind. It would be compact, lightweight, and in order to allow a carry mechanism which would allow the crank to be turned only one way, it would use an internal process of complementary arithmetic for subtraction. Then came Hitler. In 1943 Herzstark found himself arrested and imprisoned in the Buchenwald concentration camp. There his engineering skill was noticed and he was placed in a small technical camp to manufacture components to assist the Nazi war effort (in particular, parts of the V I and II missile bombs).
While Curt Herzstark had been imprisoned at Buchenwald, the Germans had retreated from Italy and whilst doing so had seized some office machines of which two truckloads were delivered to the camp. After Curt unloaded them one of the local factory owners came over to inspect them. He turned out to be Fritz Walther, the son of Curt’s father’s competitor. The Walther company was now back to making weapons for the war effort. But Walther recognised Curt Herzstark and later told the Camp Commandant of Curt’s high skills and background. Soon after his supervisor, Herr. Munich, called Curt over. Curt recounts the conversation as:
Curt Herzstark’s role gave him a relatively protected status and he survived to 1944 when the camp was liberated by US troops. His calculator plans drawn up in pencil, complete with all dimensions and tolerances were completed just as the war ended. He developed the prototypes with the Rheinmettalwerk typewriter and calculator factory which was still operating near Weimar where he was named a Director. However for a range of reasons after much exploration he agreed to a proposal by the Prince of Liechtenstein to produce the calculators there at a company established for the purpose which was named Contina AG. The financial arrangements did not live up to their promise, but because he owned the patents he was able to negotiate what was in the end a satisfactory outcome.
The first model (the Model 1) began production in 1947. Below left is a (rare) example of the Model I Curta. It still has the pin sliders which were soon to be improved upon and is in mint condition. It was made in ~July 1948, the year after production began, and is in the first 5,500 made. As can be seen the sliders are pins which were used by Curta before he introduced bakelite handles on them. Centre is a later Curta from 1967 complete with the original box in which it was sold and its instructions, all in mint condition. On the right is a Curta Model II from 1962. The Model II was a larger machine capable of a number input to 11 significant figures, compared with the more compact Model I which could accept a number accurate to 8 significant figures. Note the very ‘modern’ anodised aluminium sliders on the Model II which comes also with an optional leather carry case (not shown here).
On the Curta above, left we see the clearing ring protruding. Bringing all numbers to zero is achieved by lifting the top section and turning the clearing ring. Adding is achieved by setting successive numbers and turning the crank through a full turn. Ingeniously, pulling the crank up sets it for subtraction. Multiplication is done in the usual manner of multiplying by each successive integer of the multiplier with the upper register being advanced for each one by lifting the top section and rotating it one notch. Division is by the usual method of long division by repeated subtractions. Once more, for each partial product the operator must either correctly judge the correct number of times to turn the crank, or if an overflow is forced, reverse the operation by pushing in the crank and turning it one full turn. In short it is an excellent machine for addition and subtraction, but involves the normal acquired skill, and tedium, required by multiplication and division in any of the mechanical calculators not equipped to do it automatically.
These machines thus constitute the pinacle reached in the development of the personal hand-operated mechanical digital calculator - able to carry out all four functions by means of a crank operated machine of exquisite miniaturised workmanship and design. Production ceased in November 1970 although sales continued through 1973. At least 150,000 of the various calculator models were made.35 For more on the Curta see the marvellous collection of documents and simulations at The Curta Caclulator Page.36
4. The quest for direct multiplication and division
An entirely different evolutionary path (mentioned earlier) attempted to solving the problem of mechanising the four arithmetic operations (+, -, x, /). The emphasis was on finding ways to directly perform the more difficult two operations of multiplication and division. The approach was a development from Napier's rods - or "bones" (developed by John Napier (1550–1617). As already mentioned calculational approaches had been designed around these principles by Wilhelm Schickard in 1623, Charles Cotterel in 1667, Gaspard Schott in 1668, and Samuel Morland between 1625 and 1695.
The earliest of these machines had been that of Schickard (below, left). In the upper part is a set of rotatable Napier’s rods revealed by windows to give partial products. In the lower part is the world’s first known stylus operated adding machine to add these partial products up. A late and unique expression of these in this collection (below, right) is Justin Bamberger's Omega Calculating Machine (1903–6). In the upper section is a set of Napier’s bones revealed by moveable windows for discovering the partial products of two multiplied numbers. In the lower section is a Locke adding machine for adding them up.
The earliest of these machines had been that of Schickard (below, left) which was invented in 1623. As described earlier, it used a set of rotatable Napier’s rods in its upper part to yield partial products of the multiplication of two numbers, whilst in its lower part was the world’s first known stylus operated adding machine which could be used to add the partial products up. A later and unique expression of the same principles can be found in this collection (below, right). It is Justin Bamberger's Omega Calculating Machine (1903–6). In the upper section is a set of Napier’s bones revealed by moveable windows for discovering the partial products of two multiplied numbers. In the lower section is a Locke adding machine for adding them up.
 |  |
| 1623: Re-creation of | 1904–1905: Bamberger's Omega |
| Wilhelm Schickard’s machine | Calculating Machine |
| (collection Calculant) | (collection Calculant) |
Bamberger’s Omega used linear strips rather than the rotatable mechanisms in the upper and lower sections of the Schickard calculator. It also has some additional provision for storing intermediate results to assist long division, including the register on the top right, and the notebook. Otherwise the two machines are very similar in operation and both, with some considerable effort, can be used to perform all four functions of arithmetic. The fact that neither took off in the market place may be in part a factor of their difficulty of use and part a lack of adequately determined marketing.
A much heavier and complex mechanical approach was also explored. First it was embodied in Léon Bollée's calculating machine which won a gold medal at the Paris Exposition of 1889. One surviving example of this bulky but beautiful machine can be seen at the Musée des Arts et Métiers in Paris. This collection has only an article on this "New Calculating Machine of very General Applicability" from the Manufacturer and Builder of 1890, see below.
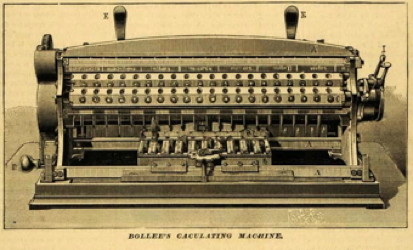
Leon Bollée Calculating Machine “A New Calculating Machine of very General Applicability" |
Similar principles were however utilised by Otto Steiger in Switzerland who in 1895 patented a rather more practical "Millionaire calculating machine" which had a simple enough mechanism to enable production on a commercial scale (see below).

1912: Millionaire Calculating Machine |
The Millionaire calculating machine combines the idea of the physical embodiment of multiplication tables with that of the proportional rack. It is able to interrogate a multiplication table represented by metal rods, and in a single crank of the handle multiplies the multiplicand by a number set between 1 and 9 then advancing its internal carriage one place ready for the next multiplier to be set and applied. Thus, for example, to multiply 4689 x 2568 an arithmometer or pinwheel would take 21 cranks of the handle (8+6+5+2) whereas the Millionaire could achieve the same outcome with only four cranks of the handle. In the lid was a set of tables of factors to assist division, a brush to keep the machine clean, and a special bolt so when being transported the carriage was held clamped in place, since if the machine were dropped the carriage was heavy enough to punch through the end of the case.
The deck of the Millionaire in this collection is shown below with the selector on the left which picks the multiplying factor, and the selector on the right which sets it for addition, subtraction, multiplication and division (AMDS). The ten sliders for setting the number to be operated on are obvious, as are the result windows, and on the far right, the crank handle.

Deck of the Millionaire Calculating Machine |
Manufactured by H.W. Egli, some 4,655 Millionaires were sold between 1895 and 193538 at a 1912 price of about US$48039 (about $11,700 in 2013 US dollars40). The Millionaire calculating machine in this collection was manufactured on 16 October 1912,41 and was until 1954 held by the B. B. Company in New York, NY. It is rather rare since it is capable of greater accuracy - 10 column - than the more common 8 column ones. The machine still operates reliably after more than 100 years.
The Millionaire was reliable, but heavy (37 kg or 81 pounds) and expensive. It could produce an answer to 20 significant figures (100 billion billion). It came in various configurations, including with a keyboard mounted on top to drive the sliders. Its value to the user depended on whether long multiplications and divisions were central to the work to be done. Styled in a rugged ‘no nonsense’ industrial design it was adopted by scientists who swore by it, railway and telegraph companies, government treasuries, and other technically oriented companies and agencies.
5. Harnessing electricity
Electricity could be utilised in appliances once it was available through an electricity grid. In the US, the first electrical supply was constructed in 1882 for lighting, with 85 customers. Electrification spread over subsequent decades, primarily in the big cities through private power companies in the first two decades of the C20. In 1926 in the UK separate electricity grids began to be connected into a national grid. It was not surprising therefore that this period of the early C20 was conducive to the introduction of electric motors to many purposes, including adding machines.
The electric motor marked the beginning of the end for all forms of mechanism more ingenious than those depending on the simple minded operation of addition and its inverse, subtraction. The greatest gains in efficiency could be obtained by simply increasing the speed with which these operations were repeated and controlled. Speed gains followed from simpler rather than more complex basic mechanisms. The control mechanisms that utilised these simple repeated basic operations, however did become more complex in the interests of using them to produce more complex and accurate outputs.
Samuel Herzstark (1867–1937), the father of Curt Herzstark who built the Curta, also was a pioneer in calculator construction and together with Gustav Perger established the Austria Calculator Machines Manufacturing Company in Vienna in 1905–6. In an interview in 1987 Curt Herzstark reports that in 1907 Samuel became the first to attach an electric motor to an arithmometer, which he equipped also with a keyboard. Curt also notes that this machine was equipped with automatic division.42 However after the 1914–18 War Herzstark returned to a demolished business. He restarted with a combination of importing and selling calculators from other manufacturers, assembling old stock of his own design, and then as the business built up designing new machines. Below is an arithmometer in this collection branded by Samuel Herzstark from 1929. It is actually a Badenia (manufactured by the German Company Math. Bauerle in the Black Forest) which Herzstark was at that time re-badging and selling.

~1929: "Herzstark" electric Calculating Machine serial 6549 |
This calculator features its original electric motor, still in good working order, and with a keyboard instead of sliders for input. The features of it were not only that it had a keyboard for input, but also a control mechanism consisting of a column of keys which enabled a number to be entered and then added through 0 to 8 repeats representing multiplication by one to nine. A view of the mechanism of this machine is shown below .
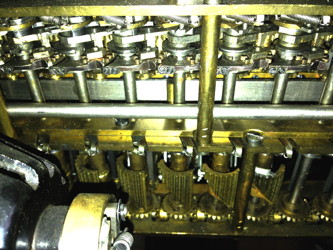
1950s-1960s: Underneath view of the Herzstark mechanism |
In the left lower corner of the above can be seen the motor, now coupled to the characteristic step drums of an arithmometer (bottom center and right), with the carry mechanism above. This puts paid to any simple story of the linear development of innovation in the calculator. Here the most modern device of the motor is being coupled to the longest serving commercial system of an arithmometer.
Whilst the above was an obvious innovation, the clumsiest approach in all the calculating devices - from the first arithmometer through to the Millionaire was division, which could only be done by a process along the lines of that done in long division. That is, the number to be divided (the dividend) is considered sequentially from the highest power of ten, and thus decomposed into a series of partial products of the successive parts of the dividend with the divisor.
In the Millionaire the outcome for each partial product could be achieved by setting the correct number in the divisor with the selector. In the Herzstark arithmometer a key column to the far right (black keys, barely visible) allowed the operator to set an addition or subtraction to repeat up to 9 times giving the same effect as with the Millionaire. Thus, although Egli and Co. did fit a motor to their Millionaire, once a motor was available with this rudimentary control mechanism, the advantage posed by the complex and heavy mechanism of the Millionaire was largely lost.
As already mentioned, both the MADAS arithmometer (and Mercedes-Euklid rack calculator) had utilised the invention by Rechnitzer to allow an overflow to be sensed and addressed by the mechanism so that division could be carried out fully automatically. With progressive further modifications, including the insertion of an electric motor, the MADAS became the highly effective electric mechanical calculating machine whose use continued right into the 1960s.

1950s-1960s: MADAS Model 20BTG serial 94046 |
The late MADAS 20BTG calculator, seen above , which is part of this collection represents a classic exemplar of the late stages of this evolutionary development. Now fully utilising an electric motor it could fluently perform all the operations of arithmetic, complete with automatic clearing and moving the carriage entirely automatically as necessary. It could also be further extended to automatically extract square roots. Its mechanism was also adopted in its essentials, then with many innovations (but with less of the Swiss robust construction of the Madas), in the US Frieden calculators. As it turned out, this final class of machines represented the pinacle of achievement in motorised mechanical calculation.
6. The Vanishing point: solid state electronics and the arrival of the HP35
Another means of performing arithmetic had largely been neglected for calculators. It had already been employed in the enormous computers that had developed from earlier work by Babbage and Scheutz. They had devised remarkably complex special purpose “difference engines” for calculating logarithms. It had also been utilised in later work by Turing and others giving rise to the electronic computing machines developed in the second world war for decoding. This was the use of binary arithmetic. The electronic version initially used valves to control on/off electric circuits each representing a single binary digit (or bit). 1 was represented by 1, two by 10, three by 11 and so on. It has been recognised since Leibniz that arithmetic could be done with these (since they represented numbers). Indeed - the method is in retrospect obvious. 10 + 01 = 11. 11+01 = 100, etc.
Using switches it was therefore possible to build a very efficient calculating machine. Valves were too bulky, energy consuming, and unreliable for a consumer device but prior sales of mechanical calculators had by now established a massive potential market. The invention of the transistor in 1947 at Bell Telephone labs, based on the quantum properties of crystals, laid the way for “solid state” electric switches at tiny scale, able to be turned on and off by one another. Light emitting diodes (LEDs) - another solid state device which emitted light when electrons having been forced into a higher energy (“excited”) state fell back to their stable energy - began to appear as practical output devices in 1962.
The first calculators to use solid-state electronics in desktop form were the ANITA VII and VIII calculators launched simultaneously in 1961, too early to use LEDs, and using instead vacuum tube displays. A variety of desktop four function calculators followed. But these could not replace the portable slide rules and other mathematical aids that were still in use in parallel with the large mechanical desktop calculators that these electronic machines began to replace.
It was in July 1972, that the old ways for scientists and citizen alike were definitively undermined. In that month, to some astonishment, the Hewlett Packard company produced the HP-35 electronic ‘pocket’ calculator (see below). It was an extraordinary leap forward, equipped not only with a a red LED display, and showing smooth performance of the arithmetic functions but also with provision to calculate reciprocals, powers, square roots, logarithms and anti-logarithms (base e and 10), the trigonometric functions, pi, and a system of registers which enabled chain operations without having to write down intermediate results.
For those of a technical orientation who could afford it, all else was now as obsolete as gaslight. Hewlett Packard followed quickly with the HP-45 appearing in the following year with a configurable display, more functions and registers, a variety of constants, and a more compact shape, more suited for the male shirt top pocket. A year later in 1974 the HP-65 was launched with all that could be done by the HP-45 but with the added feature of being user-programmable through a small built in magnetic strip reader. With that the diminutive HP calculator had taken a huge step towards the first mass-marketed personal computers (the TRS-80 - the author wrote his second book on one of these in 1981 - launched by Tandy, and the Apple-II by Apple, both launched in 1977).
The launch of the HP-35 marks what might be called the “First Vanishing Point” - the point at which all the arithmetic operations and common scientific functions required for calculation became available in a single small electronic calculator, capable of being carried on a belt or in a shirt pocket (albeit in serious peril of it falling out when one bent over to pick something up). Electronic calculators now proliferated throughout the developed and developing world displacing the rigours of mental arithmetic and caught in an ‘arms race’ to make them smaller, more powerful, easier to use, and find a niche in a highly competitive and very cluttered market.
Forty years later, in 2012, electronic solid state calculators could be found in their billions across the world. By then what might be labelled the “Second Vanishing Point” - the point at which these electronic calculators began to disappear, displaced now by “virtual calculators” encoded in the software of desk and lap-top computers, tablets, smart phones, and much else was coming into in sight (although probably had not yet arrived). But from that vantage point, even the stand-alone electronic calculators, might soon begin to follow their mechanical predecessors of two decades before fading into the misty light of receding memory.
7. In Lieu of a Conclusion
One feature of this account is it is built around a collection of historical objects. It is reasonable to ask why collect them? Why not just build it based on the great diversity of photographs of these objects in books, journal articles and on the web. The technologies are well described elsewhere in much more mechanically detailed websites. So why bring in the collection?
One reason for collecting is a banal one - the desire to collect and own things of rarity, and of course the thrill of the chase in finding, identifying and acquiring them. But beyond that it is possible for the objects to convey insight. The objects embody stories of innovation which can raise multiple questions. For example, why at some particular moment were these particular objects invented? And at a particular time what was it that allowed some, but far from all of the objects, once invented, to be taken up in use? In particular why did some get established in actual and widespread use? What limited so much more sharply the success of others? Certainly not all the answers to these sorts can be found here. But it is possible to make some potentially useful observations.
Of course, we have not considered even all the available information. There is the story of the detail of the evolution of the mechanism of the bewilderingly wide array of calculators that were built, which at best has been broadly gestured at here. Even so, perhaps enough has been said to indicate that many good ideas have waited around for their moment to be realised rather than simply the whole being driven by discovery. Much could have happened after Schickard and Pascal, but it took centuries for the various devices to become widely useful. Of course, as discussed, the device of Schickard had a potential audience in the restricted group of natural philosophers (in particular astronomers) with whom he communicated. Pascal found his machine more used as a curiosity amongst the aristocracy to add prestige, than used to add up money which was its inspiration.
There is another important consideration in relation to these sorts of innovations. An encounter with these devices suggests that that it is not possible to fully understand them, including their limitations and potential, without actually using them. A trip to, say, one of the great technology museums (for example, CNAM in Paris) will tell you how limited the experience is of, for example, seeing a Pascaline, or reading an essay about it. It is a very different experience to try to calculate with one. This leads to a rather interesting connection between understanding these technologies retrospectively, and the reasons that led to their development in the first place. For the success of that development depended on whether people learned to use them. In short this is a history not only of mechanism but of learning how it can be used.
This leads to the following observation: to learn how to use a calculating technology is not just a matter of understanding its concept. It also requires the acquisition of a type of knowledge which Jean-François Gauvin43 refers to as “gestural”. Gestural knowledge is the embodied knowledge that artisans rely on when they execute work of high craft skill. The first time someone tries to use a Thomas de Colmar arithmometer learning how to add and subtract on it efficiently takes some time. Doing division and multiplication is much more challenging, especially if attempted without a good knowledge of how it can be done on paper. The first time is slow and subject to mistakes. It is only after repeated practice that the gestures required become so practiced that they are quick, instinctive and reliable. It is only then that the potential for calculation with such a device can be can be judged. And it would only have been after this sort of practice that one could credit the Gentleman’s Magazine of 1857 claim that:
As mentioned earlier (in Part 1), gesture and mind are interconnected. When we ‘learn’ a gesture, recent neurological research demonstrates that our brains are growing new connections, in a sense rewiring, to accomodate that as what becomes a ‘habit’. Therefore, the use of the technology changes our minds. So there is a co-evolution between minds and the combination of the technology and what is required to use it successfully (sometimes referred to as ‘technique’).
The history of the technology has been one of a competition between old habits, preparedness to learn new technique, and perceived need to do so. It is not easy to throw off old successful technique and replace it with the hard acquired new approaches. Part of the success of a technological innovation is thus likely to depend on the extent to which social forces may encourage through benefit, or require through necessity, the new learning required to use it. Most new inventions are of course promoted with claims that the benefits for user or employer will outstrip the costs of change. Not infrequently these benefits might be initially overstated. Thus for the arithmometer the Gentleman’s Magazine claimed:
These overblown claims are reminiscent of similar claims for their inventions by Pascal (with this machine the user can do with ease, “without effort of memory” and “without even thinking of it”, every possible arithmetic operation46) and Moreland (allowing addition and subtraction “without charging the memory or disturbing the mind47). They show the hope, that the mental activity could be replaced by the machine. But in reality, for all of these machines it was not just a question of the high cost of obtaining it, but the learning and practice required to use it.
The Pascaline and Moreland’s inventions may have served their inventors in a range of ways, but it was not necessarily to find a broad market for them. The learning required to use it was too great, and the benefit too little in relation to existing technique. As noted earlier, even Thomas de Colmar’s arithmometer and its early successors remained on the edge of this balance. Adding and subtracting could be quickly achieved, but then it was very expensive and not necessarily any faster than doing the job on paper. The appeal thus remained quite limited, not only because of the economic price to be paid (which was high), but also the cost of acquiring the necessary embodied skill to render them genuinely superior to existing customary practice.
What goes for the Thomas arithmometer, in this sense, goes also for every calculational technology, from counting on one’s fingers, to the use of calculi on a counting board, or calculating with the abacus, the Pascaline, the Millionaire, logarithms, the MADAS, or any candidate for what was the most sophisticated mechanical calculator. Indeed even the HP-35 and HP-45 required a facility to do arithmetic backwards from the usual by its reliance on a method known as Reverse Polish. Thus for each technological development, whether Troncet or Omega, for it to find successful users each needed to be understood, and not only intellectually, but equally importantly, the potential users needed to be able to incorporate it into their embodied capacity, with each required gesture becoming so automatic as to require no or little thought.
The achievement of the late mechanical calculators (such as the MADAS and the Euklid-Mercedes) was that they greatly simplified what needed to be learned by their operators in order to achieve an efficient performance of all four arithmetic operations. But these calculators achieved this only at considerable economic cost. The Comptometer was really best for addition and (with practice) subtraction. With its key input it was fast, and its simple design was amenable to cheaper construction and mass-production. So it found a different and expanded market in the rapidly expanding commercial and government organisations of the C20. It has been said and is probably true that its inventors and promoters, Felt and Tarrant, were probably the first people in the world to become truly wealthy from the invention, production and sale of calculators.
In the above sense the history of calculation technology can be characterised not so much as the progress of mechanical invention, as it is sometimes presented, but as a more subtle evolving relationship between mind, body and material artefacts or put another way as an interaction between evolving technology, history, culture, mental skills, social capacities and aspirations. The search for a successful innovation was a strange mix of finding a place where these aspects converged to make the innovation seem useful, and at the same time not only economically but also culturally accessible. The requirement to change was not just set in the machine, but also in the humans who made up the society.
The final conquest of the electronic calculator occurred when literacy, design, familiarity, cost and perceived need coincided to sweep all else before it. Never had the market been more prevalent in the negotiation of daily life. Science and technology now dominated every corner of the developed world and was making rapid inroads elsewhere. Literacy was at its highest in the developed world with arithmetic education now a requirement for every child in the extended period of compulsory schooling. Money promised was supposed to free time. Technology was now widely accepted as being the answer to drudgery. Further, there was a growing social acceptance of life-long change, and an emerging concept of the desirability of ‘life-long learning’. The new consumer calculators were increasingly cheap, required little knowledge to use, displaced the mental effort of recall of multiplication tables and mental and written arithmetic. Printers attached to them produced now comparatively permanent records. And the whole increasingly seamlessly fitted a world which would soon be interconnected through computers and telecommunications into an ever more pervasive communications web. Calculators had not only reached a desired end. They also had found their moment when that end was widely required.
One might ask if there is any lesson in this for the future. Clearly the technology of calculation is now passing not only the first but perhaps even towards the second vanishing point where it converges and merges with other electronic devices which themselves have become so much part of the habitude of daily life, especially in the developed world, that their presence is sinking into the invisibility of the routine environment of human experience. But in doing so much of habit had to be relearned, and in the consequence human thinking, as well as collective culture has transformed.
This is of course a history of one area of innovation. So it may have some relevance to other areas of innovation in the world in which we now live. It is perhaps appropriate to remember that humans have reached the point where their innovation is actually destabilising the physical world in which they live - a situation unimaginable for most of the time in which the developments discussed here have taken place. But it is the case that humans are now challenged with the requirement to achieve an unprecedented level of innovation if the planet is to remain stable. The story of innovation in calculators tells us that whilst the time may be ripe for us to accept a great deal of change, it will require re-learning to be comfortable with the many innovations that will be needed to achieve it. Getting there will require people to re-learn and reshape many attitudes, hidden assumptions and habitual ways of living. In seeking to make those changes, we might reflect on the many challenges overcome in the simpler long history of how humans have learned to calculate, and what that means for the learning and innovation that will now be required.
« Part 2 The Modern Era | | »
1 For more on this see Camilleri and Falk, Worlds in Transition, pp. 134–44. (↑)
2 Werner Heisenberg, The Physicist’s Conception of Nature, Hutchinson Scientific and Technical, London, 1958, p. 11. (↑)
3 see Bruce O.B. Williams and Roger G. Johnson, “Ready Reckoners”, IEEE Annals of the History of Computing, October-December, 2005, pp. 64–80. (↑)
4 M.N. Wise, The Values of Precision, Princeton University Press, USA, 1995, p. 318. (↑)
5 An exception is the ingenious MONIAC hydraulic computer used to demonstrate macroeconomic theory. See for example, Anna Corkhill 'A superb explanatory device' University of Melbourne Collections, issue 10, June 2012 (↑)
6 See for example, A.P. Kidwell, “'Yours for Improvement'—The Adding Machines of Chicago, 1884–1930”, IEEE Annals of the History of Computing, July-September, 2001, pp. 3–6. (↑)
7 Patent no. 1420, 18 November 1820 (↑)
8 For the most authoritative website on the Thomas de Colmar machines and history see Valéry Monier’s magisterial site http://www.arithmometre.org, viewed 20 July 2013. (↑)
9 see Friedrich W. Kistermann, “When Could Anyone Have Seen Leibniz's Stepped Wheel?”, IEEE Annals of the History of Computing, Volume 21, Number 2, 1999, pp. 68–72. However, as Stephen Johnston (see reference below) points out, images of Leibniz’s machine were available from the late C18, see for example 1744 engraving in Annegret Kehrbaum and Bernhard Korte, Calculi: Bilder des Rechnens einst und heute (Images of Computing in Olden and Modern Times), Opladen, 1995, p. 61. (↑)
10 Franz Reuleaux, Die Thomas'sche Rechenmaschine, Freiberg, 1862 reproduced in Reuleaux's article in Der Civilingenieur, 8, 1862, and reproduced in turn by Johnston, “Making the arithmometer count”, figure 2. (↑)
11 Stephen Johnston, “Making the arithmometer count”, Bulletin of the Scientific Instrument Society, Volume 52, 1997, pp. 12–21. (↑)
12 Peter Borscheid, “Europe: Overview”, in Peter Borscheid and Niels Vigo Haueter (eds), World Insurance: The Evolution of a Global Risk Network, Oxford University Press, Oxford, 2012, p. 39. (↑)
13 ibid. (↑)
14 As cited by Johnston, Jean Marguin, Histoire des instruments et machines à calculer, Paris, 1994, p. 111: 'Pendant un demi-siècle, la machine y régna seule.' (↑)
15 Johnston, “Making the arithmometer count”. (↑)
16 Brunel to Adams, 18 March 1866, Bristol University Library, Brunel Collection, Letter Book VII, f. 106, cited in Johnston, ibid. (↑)
17 Calculated using the http://www.measuringworth.com calculator on 21 July 2013 (↑)
18 ibid (↑)
19 P. A. Kidwell, “American Scientists and Calculating Machines- From Novelty to Commonplace”, Annals of the History of Computing, Volume 12, Number 1, 1990, pp. 31 (↑)
20 See Ernst Martin, The Calculating Machines (Die Rechenmaschinen): Their History and Development, ed. and trs. by Peggy Aldridge Kidwell and Michael R. Williams, MIT Press, Mass., 1992, p. 191–4 (↑)
21 ibid. p 198. (↑)
22 US Patent 809075, Filed by Alexander Rechnitzer of Viennea, US Patent Office, 29 June 1901, granted 2 January 1906, page 9, clauses 80–110. For a more comprehensive set of his patents see http://www.rechnerlexikon.de/en/wiki.phtml?srch=rechnitzer&title=Spezial%3APatentpage viewed 25 July 2013. (↑)
23 Martin Reese, Hamburg, 55 erfolgreiche Jahre: MADAS-Rechenautomaten aus der Schweiz 1913 - 1968, http://www.rechnerlexikon.de/files/MADAS—8-2010.pdf, viewed 18 February 2012. (↑)
24 Diana H. Hook and Jeremy M. Norman, Origins of Cyberspace, Novato, California, 2002, p. 255. (↑)
25 ibid (↑)
26 Hook and Norman, Origins of Cyberspace, p. 255. (↑)
27 “Walther Company History”, http://www.carl-walther.de/cw.php?lang=en&content=history, viewed 23 July 2013. (↑)
28 Ray Mackay, “The Walther Company: Historic Recollections”, December 1997. http://www.xnumber.com/xnumber/walther.htm, viewed 22 July 2013. (↑)
29 John Wolff, “John Wolff’s Web Museum: Walther Calculators Overview” http://home.vicnet.net.au/~wolff/calculators/Walther/Walther.htm, viewed 23 July 2013. (↑)
30 Martin, The Calculating Machines (↑)
31 http://www.rechnerlexikon.de/en/artikel/Spezial:Allpages (↑)
32 http://home.vicnet.net.au/~wolff/calculators/ (↑)
33 ‘/Origins of Cyberspace, p. 244. (↑)
34 An Interview with Curt Herzstark, OH 140, conducted by Erwin Tomash on 10–11 September 1987, Nendeln, Liechtenstein, (English Translation). (↑)
35 An Interview with Curt Herzstark (↑)
36 The CURTA Calculator Page, http://www.vcalc.net/cu.htm, viewed 25 July 2013. (↑)
37 1890: Leon Bollée Calculating Machine, "A New Calculating Machine of very General Applicability", The Manufacturer and Builder, July 1890, p. 156. Original print article. (↑)
38 Origins of Cyberspace p. 242. (↑)
39 Price list in Edmonds Collins, The Millionaire Calculating Machine, pamphlet, Edmond Collins, 35 Dearborn St, Central Chicago, ~1912. See also price of US$500 at 1914 prices given by Luc de Brabandere cited in B.O.B. Williams,” Check Figures—A Once Ubiquitous Tool for Book-keepers, published in Slide Rule and Calculation monographs, Slide Rule Circle, UK, 2002, pp. 59–98. (↑)
40 Calculated using the http://www.measuringworth.com calculator on 24 July 2013 (↑)
41 Letter from H.W. Egli Ltd, Zurich, dated 20 November 1967 (collection Calculant). (↑)
42 An Interview with Curt Herzstark, OH 140, conducted by Erwin Tomash on 10–11 September 1987, Nendeln, Liechtenstein, (English Translation). (↑)
43 Jean-François Gauvin , Habits of Knowledge: Artisans, Savants and Mechanical Devices in Seventeenth-Century French Natural Philosophy, The Department of the History of Science, in partial fulfillment of the requirements for the degree of Doctor of Philosophy in the subject of History of Science, Harvard University, Cambridge, Mass., USA, November 2008, pp. 113. (↑)
44 The Gentleman’s Magazine, Volume 202, “The monthly intelligencer”, January 1857, p. 100, http://books.google.fr/books?id=Rf0IAAAAIAAJ&pg=PA100&dq=arithmometer&as_brr=1#v=onepage&q=arithmometer&f=false, viewed 23 July 2013. (↑)
45 ibid (↑)
46 Pascal, ligé de retenir ou d'emprunter les nombres nécessaires, et combien d'erreurs se glissent dans ces rétentions et emprunts, à mois d'une très longue habitude et, en outre, d'une attention profonde et qui fatigue l'esprit en peu de temps. Cette machine délivre celui qui opère par elle de cette vexation; il suffit qu'il ait le jugement, elle le relève du défaut de la mémoire; et, sans rien retenir ni emprunter, elle fait d'elle-même ce qu'il désire, sans même qu'il y pense." p. 337 (↑)
47 S. Moreland, A New and Most Useful Instrument for Addition and Subtraction of Pounds, Shillings and Pence 1672, title page. (↑)
« Part 2 The Modern Era | | »
Pages linked to this page
 This work by Jim Falk is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License Click on the logo to the left to see the terms on which you can use it.
This work by Jim Falk is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License Click on the logo to the left to see the terms on which you can use it.